Đồ thị hàm số \(y = \dfrac{{3x + \sqrt {{x^2} + 1} }}{{2 + \sqrt {3{x^2} + 2} }}\) có bao nhiêu đường tiệm cận ngang?
Hãy suy nghĩ và trả lời câu hỏi trước khi xem đáp án
Lời giải:
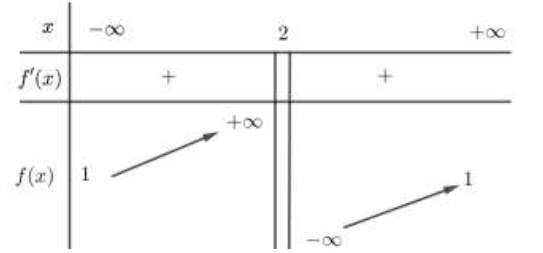
Báo saiTXĐ: \(\mathbb{R}\).
Ta có:
+) \(\mathop {\lim }\limits_{x \to + \infty } y = \mathop {\lim }\limits_{x \to + \infty } \dfrac{{3 + \sqrt {1 + \dfrac{1}{{{x^2}}}} }}{{\dfrac{2}{x} + \sqrt {3 + \dfrac{2}{{{x^2}}}} }}\)\( = \dfrac{4}{{\sqrt 3 }} = \dfrac{{4\sqrt 3 }}{3}\)
+) \(\mathop {\lim }\limits_{x \to - \infty } y = \mathop {\lim }\limits_{x \to - \infty } \dfrac{{3 - \sqrt {1 + \dfrac{1}{{{x^2}}}} }}{{\dfrac{2}{x} - \sqrt {3 + \dfrac{2}{{{x^2}}}} }}\)\( = - \dfrac{2}{{\sqrt 3 }} = - \dfrac{{2\sqrt 3 }}{3}\)
Suy ra đồ thị hàm số có các tiệm cận ngang: \(y = \dfrac{{4\sqrt 3 }}{3}\) và \(y = - \dfrac{{2\sqrt 3 }}{3}\).
Câu hỏi liên quan
-
Cho hàm số \(y=f(x)\) có bảng biến thiên sauKhẳng định nào sau đây đúng
-
Đồ thị của hàm số nào dưới đây không có đường tiệm cận ?
-
Đồ thị hàm số \(y=x-\sqrt{x^{2}-4 x+3}\) có tiệm cận ngang là
-
Số tiệm cận của hàm số \(y=\frac{\sqrt{x^{2}+1}-x}{\sqrt{x^{2}-9}-4}\) là
-
Đường thẳng y=-2 là tiệm cận ngang của đồ thị hàm số.
-
Cho hàm số \(y=\frac{x-1}{x+m}(m \neq-1)\) có đồ thị là (C) . Tìm để đồ thị (C) nhận điểm I(2;1) làm tâm đối xứng.
-
Cho hàm số y=f(x) có bảng biến thiên như sau:
Tổng số đường tiệm cận đứng và tiệm cận ngang của đồ thị hàm số đã cho bằng -
Cho hàm số \(y=\frac{x^{2}+2 x+3}{\sqrt{x^{4}-3 x^{2}+2}}\) Đồ thị hàm số đã cho có bao nhiêu đường tiệm cận?
-
Cho hàm số \(f(x)=\frac{a x+1}{b x+c}(a, b, c \in \mathbb{R})\)có bảng biến thiên như sau:
Trong các số a b , và c có bao nhiêu số dương?
-
Với giá trị nào của m, đồ thị hàm số \(y = \frac{{x + 1 - \sqrt {{x^2} + 3x} }}{{{x^2} + \left( {m + 1} \right)x - m - 2}}\) có đúng hai đường tiệm cận?
-
Cho hàm số \( f\left( x \right) = \frac{{ax + 1}}{{bx + c}}\) (a,b,c thuộc R) có bảng biến thiên như sau:
Trong các số (a,b ) và (c ) có bao nhiêu số dương ?
-
Đồ thị hàm số \(y=\frac{x-2}{x^{2}-4}\) có mấy tiệm cận?
-
Tìm tất cả các giá trị thực của tham số mm để đồ thị hàm số \(y = \frac{{x - 1}}{{x - m}}\) có tiệm cận đứng.
-
Tiệm cận ngang của đồ thị hàm số \(y=\frac{x-20221}{x+2020}\) là
-
Cho hàm số y=f(x) có bảng biến thiên như hình dưới đây
Tổng số tiệm cận ngang và tiệm cận đứng của đồ thị hàm số \(y=\frac{1}{2 f(x)-1}\) là?
-
Cho hàm số bậc ba \(f(x)=a x^{3}+b x^{2}+c x+d\) có đồ thị như hình vẽ bên dưới:
Hỏi đồ thị hàm số \(g(x)=\frac{\left(x^{2}-3 x+2\right) \sqrt{x-1}}{(x+1)\left[f^{2}(x)-f(x)\right]}\) có bao nhiêu đường tiệm cận đứng?
-
Cho hàm số y=f(x) liên tục trên \(\mathbb{R} \backslash\{1\}\) có bảng biến thiên như hình vẽ. Tổng số đường tiệm cận đứng và đường tiệm cận ngang của đồ thị hàm số y=f(x).
-
Cho hàm số\(y=\frac{2 x-3}{x-2}(C)\). Gọi d là tiếp tuyến bất kì của (C), d cắt hai đường tiệm cận của đồ thị (C) lần lượt tại A, B. Khi đó khoảng cách giữa A và B ngắn nhất bằng
-
Xác định m để đồ thị hàm số \(y=\frac{x-1}{x^{2}+2(m-1) x+m^{2}-2}\) có đúng hai tiệm cận đứng
-
Đồ thị của hàm số nào trong các hàm số dưới đây có tiệm cận đứng?
