ADMICRO
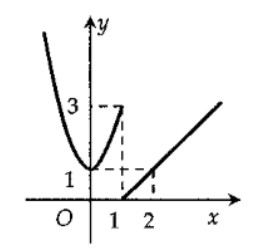
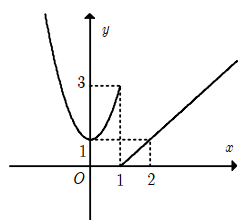
Cho hàm số . Giá trị của a để f (x) liên tục trên là
Chính xác
Xem lời giải
Hãy suy nghĩ và trả lời câu hỏi trước khi xem đáp án
ZUNIA12
Lời giải:
Báo saiTXĐ:
Với ta có hàm số liên tục trên khoảng
Với ta có hàm số liên tục trên khoảng .
Với ta có .
Để hàm số liên tục tại
Vậy a =1hoặc a = - 2 thì hàm số liên tục trên .
ZUNIA9
AANETWORK