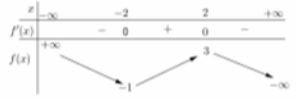
Cho hàm số bậc bốn f(x) có bảng biến thiên như sau:

Số điểm cực trị của hàm số \(g(x) = {x^4}{[f(x - 1)]^2}\) là

A. 7

B. 5

C. 9

D. 11

Hãy suy nghĩ và trả lời câu hỏi trước khi xem đáp án

Chủ đề: Đề thi THPT QG

Môn: Toán

Lời giải:

Báo sai

Đặt \(f(x) = a{x^4} + b{x^3} + c{x^2} + dx + e \Rightarrow f'(x) = 4a{x^3} + 3b{x^2} + 2cx + d.\)

Từ bảng biến thiên, ta có:

\(\begin{array}{l}
\left\{ \begin{array}{l}
f(0) = 3\\
f(1) = - 1\\
f( - 1) = - 1\\
f'(0) = 0\\
f'(1) = 0\\
f'( - 1) = 0
\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}
e = 3\\
a + b + c + d + e = - 1\\
a - b + c - d + e = - 1\\
d = 0\\
4a + 3b + 2c + d = 0\\
- 4a + 3b - 2c + d = 0
\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}
a = 4\\
b = 0\\
c = - 8\\
d = 0\\
e = 3
\end{array} \right.\\
\Rightarrow f(x) = 4{x^4} - 8{x^2} + 3,{\rm{ }}f'(x) = 16{x^3} - 16x.
\end{array}\)

Ta có:

\(\begin{array}{l}
g'(x) = 4{x^3}{[f(x - 1)]^2} + {x^4}.2.f(x - 1).f'(x - 1) = 2{x^3}f(x - 1){\rm{[2}}f(x - 1) + xf'(x - 1){\rm{]}}\\
g'(x) = 0 \Leftrightarrow 2{x^3}f(x - 1){\rm{[2}}f(x - 1) + xf'(x - 1){\rm{]}} = 0 \Leftrightarrow \left[ \begin{array}{l}
{x^3} = 0\\
f(x - 1) = 0\\
{\rm{2}}f(x - 1) + xf'(x - 1) = 0
\end{array} \right.\\
+ {x^3} = 0 \Leftrightarrow x = 0.\\
+ f(x - 1) = 0 \Leftrightarrow \left[ \begin{array}{l}
x - 1 = {t_1} < - 1\\
x - 1 = {t_2} \in ( - 1;0)\\
x - 1 = {t_3} \in (0;1)\\
x - 1 = {t_4} > 1
\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}
x = 1 + {t_1} < 0\\
x = 1 + {t_2} \in (0;1)\\
x = 1 + {t_3} \in (1;2)\\
x = 1 + {t_4} > 2
\end{array} \right..\\
+ {\rm{2}}f(x - 1) + xf'(x - 1) = 0 \Leftrightarrow {\rm{2}}f(t) + (t + 1)f'(t) = 0{\rm{ }}(t = x - 1)\\
\Leftrightarrow 2\left( {4{t^4} - 8{t^2} + 3} \right) + (t + 1)\left( {16{t^3} - 16t} \right) = 0 \Leftrightarrow \left( {4{t^4} - 8{t^2} + 3} \right) + (t + 1)\left( {8{t^3} - 8t} \right) = 0\\
\Leftrightarrow 12{t^4} + 8{t^3} - 16{t^2} - 8t + 3 = 0.
\end{array}\)

Xét hàm số \(h(t) = 12{t^4} + 8{t^3} - 16{t^2} - 8t + 3.\)

Ta có:

\(\begin{array}{l}
h'(t) = 48{t^3} + 24{t^2} - 32t - 8.\\
h'(t) = 0 \Leftrightarrow 48{t^3} + 24{t^2} - 32t - 8 = 0 \Rightarrow \left[ \begin{array}{l}
{t_1} \approx 0,7287\\
{t_2} \approx - 0,2287\\
{t_3} = - 1
\end{array} \right..
\end{array}\)

Từ bảng biến thiên suy ra phương trình có 4 nghiệm t nên có thêm 4 nghiệm x nữa.

Phương trình g’(x) = 0 có 9 nghiệm đơn phân biệt. Vậy hàm số g(x) có 9 cực trị.

Chọn: C

Câu hỏi này thuộc đề thi trắc nghiệm dưới đây, bấm vào Bắt đầu thi để làm toàn bài